Share Post:
Polynomials are a key part of algebra that many people encounter in their math studies. They consist of terms that include variables, coefficients, and exponents, and they can be combined using addition, subtraction, and multiplication.
In this article, we will explore the different types of polynomials, explain what makes each type unique, and provide examples to illustrate these concepts.
What is a Polynomial?
A polynomial is an expression made up of variables, coefficients, and exponents combined using addition, subtraction, and multiplication. Each term in a polynomial is a product of a constant and a variable raised to a whole number exponent. To understand more about whole number exponents, you might first want to explore natural numbers. Polynomials are used in various mathematical calculations and have different types based on their structure and degree.
Example: 5x^2 – 3x + 7.
Types of Polynomials Based on the Number of Terms
Polynomials can be categorized based on the number of terms they have. Here are the main types:
Monomial
A polynomial with only one term.
Example: 5x, 7, -3y.
Binomial
A polynomial with exactly two terms.
Example: 4x^2 + 3, -5x + 6y.
Trinomial
A polynomial with exactly three terms.
Example: 2x^2 + 3x + 4, x^3 – 2x + 5.
To learn more about polynomials and how they relate to other mathematical concepts, you might also want to explore the definition of a point in geometry.
Types of Polynomials Based on Degree
Polynomials can also be classified according to their degree, which is the highest exponent of the variable in the polynomial. Sequences, such as arithmetic and geometric sequences, often play a role in polynomial expressions, helping to describe number patterns and growth rates. Here are the main types:
Constant Polynomial
A polynomial of degree 0.
Example: 5.
Linear Polynomial
A polynomial of degree 1.
Example: 3x + 2.
Quadratic Polynomial
A polynomial of degree 2.
Example: 4x^2 + 3x + 1.
Cubic Polynomial
A polynomial of degree 3.
Example: 2x^3 – x^2 + 3x + 5.
Quartic Polynomial
A polynomial of degree 4.
Example: x^4 + 2x^3 – x + 1.
Quintic Polynomial
A polynomial of degree 5.
Example: 2x^5 – 3x^4 + x^3 – x + 7.
Key Properties of Polynomials
Polynomials possess several important properties that are fundamental to their behavior in mathematical operations.
Here are some of the key properties.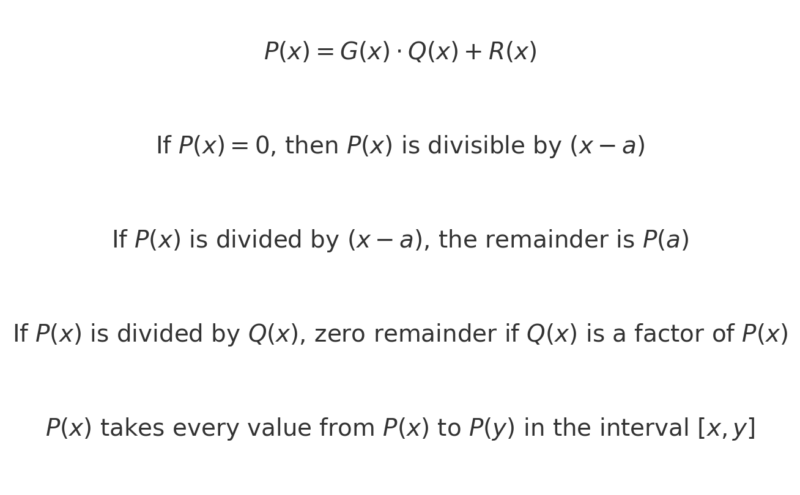
Division Algorithm
If a polynomial P(x)ย is divided by a polynomial G(x), it results in a quotient Q(x)ย and a remainder R(x), expressed as P(x)=G(x)โ Q(x)+R(x)P(x) = G(x) \Q(x) + R(x)P(x)=G(x)โ Q(x)+R(x).
Bezoutโs Theorem
A polynomial P(x) is divisible by the binomial (xโa)(x – a)(xโa) if P(a)=0P(a) = 0P(a)=0.
Remainder Theorem
If P(x)ย is divided by (xโa)(x – a)(xโa), the remainder is P(a).
Factor Theorem
A polynomial P(x) divided by Q(x)ย results in a zero remainder if Q(x)ย is a factor of P(x).
Intermediate Value Theorem
If P(x)ย is a polynomial and P(x)โ P(y)P(x) \ P(y)P(x)๎ =P(y) for x<yx < yx<y, then P(x) takes every value from P(x) to P(y)ย in the closed interval [x,y].
Conclusion
Understanding polynomials is essential for solving various mathematical problems and equations. They are classified based on the number of terms and their degree, each serving a unique purpose in mathematics.
From evaluating and adding polynomials to exploring their properties, polynomials form the foundation of algebra and have numerous applications in science and engineering.
